Order-N Technique
A Brief Description
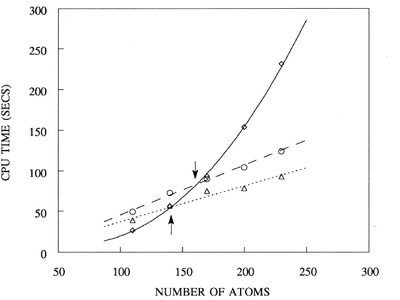Total energy calculations and molecular dynamics simulations with forces derived from reliable potentials (first-principles or tight-binding Hamiltonians) are the central techniques of many material science problems. However, the application of these to study the properties of complex systems of realistic sizes (N~10^3-10^6) has been hampered by the N^3-scaling in computational time of total energy and atomic forces. To overcome this bottleneck, we have developed an order(N) procedure where the computational time scales linearly with the size of the system. The feasibility of our approach has recently been demonstrated for the case of a non-orthogonal tight-binding hamiltonian although the main concepts under-pinning the calculation are equally applicable in the context of first-principles calculations. Using this O(N) algorithm, we have recently determined the equilibrium structure for a 1000-atom silicon cluster, structure of clean Si(100) surface and performed large scale simulations of thin film growth of silicon deposited on Si(100) surface. These studies can be used to gain an understanding of the initial stages of growth of thin films on a substrate at the atomic level. The improved understanding of the growth properties of thin films of silicon is important for semiconductor technology because successful production of electronic devices of increasing complexity and decreasing size relies on the ability to control structure down to exceedingly small scales.
Recent Publications Relating to O(N) Method
- C. S. Jayanthi, S. Y. Wu, J. Cocks, N. S. Luo,Z. L. Xie, M. Menon, and G. Yang; Phys. Rev. B57,3799(1998)